Regrouping is one of the most important concepts of second grade! Ask your student to explain the poster and examples below (they should know to trade ("regroup") 10 ones for 1 ten, as well as 10 tens for 1 hundred). This skill is the precursor for two and three-digit addition and subtraction in Chapter 4!
______________________________________________________
We've just started working on Chapter 2, which is all about working with ten! Students will learn the sums to ten and will become fluent with them - this skill will help with adding and subtracting larger numbers later. Students will also learn to compare both numbers and number sentences, as well as to add and subtract ten with fluency.
Number Line Hotel: Below is the "Number Line Hotel." This unique number line is a tool used to help students learn how to add and subtract numbers quickly. Students have learned that the "hotel" has staircases that go from the right side of the hotel up the left side of the next floor (following the numbers in sequential order), but also that there are special elevators in each "room" (number) that go straight up and down. During a lesson last week, students discovered that to add or subtract 10 quickly, you can use the elevator (see example on pink paper below). This skill will be especially useful when we start to work with larger numbers.
For example, to solve 35 + 22 = ___, students would start at 35, take the "elevator" up 2 floors (adding 20) and then walk two rooms to the right (adding 2 more). We will be using the Number Line Hotel frequently in class, especially in the first half of the year!
Ask your student to explain how it works as a way for them to review and practice!
Comparing Numbers: Students are learning to compare numbers using the symbols for greater than ( > ) and less than ( < ). One way we try remember the direction of the symbols is this phrase: "the BIG, hungry, alligator wants to eat the BIGGER snack" (and therefore the "mouth" should open toward the bigger number).
8 > 7 6 < 7
______________________________________________________
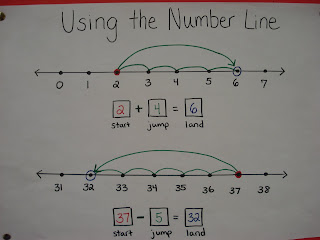
In Chapter 1 of Think Math! students will be working with patterns, skip counting, and adding & subtracting on the number line. Below are also some vocabulary and math terminology that we will be using in the classroom. Ask your student to explain what we are learning and help them to practice these concepts at home!
Repeating Patterns: patterns that have a unit that repeats over and over in
a predictable way
Examples: 2, 5, 2, 5, 2, 5, 2, 5 (pattern unit: 2, 5)
+, -, =, +, -, =, +, -, = (pattern unit: +, -, =)
Growing Patterns: patterns that grow or shrink in a predictable way
Examples: 2, 4, 6, 8, 10, 12 (add 2)
25, 20, 15, 10, 5, 0 (subtract 5)
Some problems might seem especially tricky:
1, 2, 4, 7, 11, 16 (add 1, then 2, then 3, then 4, etc.)
When presented with a pattern problem, students should first identify whether it is repeating or growing . If the pattern is repeating, they should identify the pattern unit in order to figure out what comes next. If the pattern is growing, students will need to determine if the numbers are increasing or decreasing, and then figure out by how much (checking that their rule works for the entire sequence).